Fun Science / A Brief History of Gravity

I always thought that the story behind great scientific discoveries is fascinating; and who can ignore the discovery of gravity, the very first of all discoveries that were about to come. So I decided to present my understanding on how gravity (along with astronomy) came into existence, beginning with the ancient Greeks and ending with Einstein, who summed up all previous efforts into one elegant theory.

Ancient Greece & Ptolemy

A few centuries B.C. the Greeks described the first realistic model of the universe. The Earth in the center of it, and a sphere in which the stars where fixed on was somewhere in outside. This notion explained why the stars appear in the same places each year. The sun and the moon were also moving in circles around the earth.

However, there where objects in the sky that see to wander around without any predictable type of motion; they named these objects planets, from the Greek word for wanderer. Aristotle and Plato put the planets perfectly circling the earth, and that all objects were falling onto the earth.

Although this picture nowadays seems ridiculous, one must understand the context of these times: people thought that nature must be absolutely symmetric, perfect. The only types of motion that are perfect were the straight line and the circle.

Also, earth was considered the heaviest of all 4 natural elements (earth, water, fire, air). Therefore the Earth was placed in the center of the universe so that all objects will fall towards it in straight lines. The only objects that couldn’t fall in straight lines, the planets and the stars, had to move in circles around the earth. (An astronomer named Aristarchos claimed that the sun is in the center and that the earth moves yearly around the sun and daily around its axis, but his work was never appreciated).

In the 2nd century A.D. Claudius Ptolemy codified these ideas by creating a complicated system of circles and epicycles, a combination of which could explain all types of movements observed on the sky. If an observation disagreed with this theory, another epicycle was added so that it could be explained with the objects maintaining their perfect circular motions. His book, The Almagest, was the principal astronomy textbook for over 1400 years, until Copernicus came into play.

A Priest Started it All: Nicolas Copernicus

Who would expect from a Polish priest to dare the place of the earth in the universe! Copernicus was born in 1473 and it was greatly interested in sky observations, becoming one of the most well known astronomers of his time. At the age of 41, he started giving to his friends an anonymous manuscript were he claimed that if instead of the earth, strictly as a mathematical convenience, the sun was placed in the center of the universe, then Ptolemy’s system of epicycles could be greatly simplified.

Of course he was afraid of letting his idea out because he would face extreme consequences. However throughout his life he worked secretly on this idea of his, extending his observations and refining his theory. Towards the end of his life, he decided to publish his lifetime work, On the Revolutions of the Celestial Spheres. He is said to have received finally a printed copy of his work in his deathbed, in 1543.

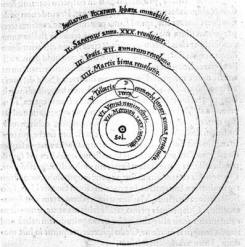
At the middle of all things lies the sun. As the location of this luminary in the cosmos,thatmost beautiful temple, would there be any other place or any better place than the centre, from which it can light up everything at the same time? Hence the sun is not inappropriately called by some the lamp of the universe, by others its mind, and by others its ruler.

Copernicus’ work was never appraised even after his death. Very few people read the book and even fewer appreciated its scientific value. However it was a small stone rolling down the hill that would eventually cause an avalanche to happen.

A Danish Observer: Tycho Brahe

Brahe was a Danish nobleman, who was born 1546, 3 years after Copernicus’ death. Interested in astronomical observations, he discovered a new star at the age of 26, which made him quite famous among astronomers. This allowed him to get financial support to build the best astronomical observatory of that time, and started mapping the sky with excellent precision (Note that the telescope was invented many years later!). However the king disliked his work and so he was forced to move to Hungary when he was 42.

Brahe did not embrace the Copernician view of the universe; he invented one of his own, by placing the earth again at the center of the universe. However the sun now circled the earth, and all the planets circled the sun. Naive as it may now seem, this was a audacious approach since it didn’t need the crystal spheres on which the planets where revolving. Smart as he may have been, he didn’t have the ability to mathematically support his theory; he needed a genius greater than his, and he found one in the face of Johannes Kepler.

And Then a Shock: Johannes Kepler

Kepler was born in 1571 in Germany and became a mathematics school teacher. He was a brilliant, intelligent guy who really liked the mathematical perfection in nature, so he really admired Plato, his ideas of perfect circular motion and the 5 perfect solids (tetrahedron, cube, octahedron, dodecahedron, icosahedron).

At the age of 24, while he was teaching geometry and was inscribing circles inside and outside an equilateral triangle, he realized that the ratio of the diameters of the 2 circles matched the ratio of the orbits of Jupiter and Saturn (almost twice one the other).

Astounded by his discovery, he picked the 5 perfect solids, nested them one inside the other, and placed spheres between them, to get a total of 6 spheres (an innermost, 4 in between, and an outermost), all inscribed inside and outside the solids. Using his mathematical talent, he kept arranging the solids in various orders, until he found a configuration for which the diameters of the spheres matched almost exactly the orbits of the 6 known at the time planets.

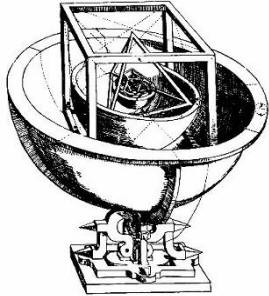
The whole thing fitted unbelievably perfectly together: 5 perfect solids and therefore 6 planets in the spheres between them. The almost impossible mathematical harmony oh his theory made him think that he had seen into the mind of the Creator. A year later, 1596, he wrote his first book Mysterium Cosmographicum, which made Brahe notice him and initiated their collaboration.

Although these two guys were opposite characters, they needed each other: Brahe needed Kepler’s talent and Kepler needed Brahe’s observational data in order to verify his theoretical structure. Unfortunately 2 years after they met, Brahe died in 1601, and so Kepler was destined to continue on his own. He then struggled to describe the motion and the orbits of the planets according to Brahe’s data. Although he could describe earth’s motion as a perfect circle, with the sun just a little bit displaced from it’s center, he couldn’t fit Mars’ orbit to any circle at all, no matter how hard he tried.

And then a real stroke of genius came: Kepler broke once and for all the tight bonds to the perfect platonic world by realizing that maybe the orbits of the planets were not perfect circles after all! In his own words:

I was almost driven to madness in considering calculating this matter. I could not find out why they would rather go in an elliptical matter rather than a circle. Oh ridiculous me!

“Oh ridiculous me!” There is no true scientist who hasn’t at least once discovered a truth like that, no matter how important or not it is, a fact that once realized seems so obvious and explains the phenomena so much better than ever before, so that everything now starts falling into place.

You must realize that Kepler’s argument was a humongous step of reasoning; for thousands of years everyone (even Copernicus and Brahe) thought that nature should behave perfect, symmetrical and harmonic. To alter this forever-established idea, now, that was insane and out of the question. Kepler was the first man who ever dared to challenge nature so greatly; and he quickly realized that this indeed must be the case. The planets should move in ellipses and not circles anymore!

Once this assumption was made, it was a matter of time before he extrapolated his 3 famous laws, which he published in two books, Astronomia Nova and Harmonices Mundi at the age of 38 and 48 respectively. Kepler’s theory along with Brahe’s data made astronomy 100 times more accurate than ever before. All I want to point out is the fact that in order for a minor experimental fact to be fitted into theory, a huge reconsideration of our understanding of the world must be introduced. That was the first time it ever happened, but it has occurred numerous times since then in the history of science.

Kepler died in 1630 at the age of 59 leaving behind him the first accurate mathematical treatment of the cosmos.

The First True Physicist: Galileo Galilei

In questions of science, the authority of a thousand is not worth the humble reasoning of a single individual.

While Kepler was busy working on his planetary motion theory, at the same time an Italian man named Galileo (born at 1564) was working on the earthly effects of gravity (of course none of them realized at the time that they were investigating the two sides of the same coin, gravity. Newton would bring this unification several years later). In fact it’s ironic that although Galileo accepted the Copernician system, he never embraced Kepler’s laws.

The Copernician system defied the common sense though: if indeed the earth is spinning on its axis and isn’t at rest at the center of the universe, then why can’t we sense the motion? If one were to let an object fall from a tower, then it shouldn’t fall directly down but a little bit to the side because of the earth’s rotation during the fall time. This distance off the axis can be easily calculated, and Galileo found it to be about half a mile!

So there was something wrong with the understanding of the world. Galileo introduced for the first time the very essence oh physics: experiments should be done (like the ancient Greeks did) but they should be tested with the theory using mathematical language. He created inclined planes of different inclination and let balls to roll on them, measuring the time it takes to go down the slope.

He quickly realized that no matter what the slope was, gentler or steeper, given twice the time a ball would travel four times the distance. At that point he made his first huge leap of imagination: that this must be true in the limiting case, even if the plane was completely vertical, so it must be true for free falling bodies too. He managed to work out the mathematics of his experiments and figured that it meant uniform acceleration.

Then as a second giant leap on imagination, he thought of bodies falling in the vacuum, an idea unthinkable at that point, for nothing to exist by itself! He broke free of the bonds of the Aristotelian thought and stated that the only reason that makes bodies fall at different speeds was the existence of air; if one were to do the experiments in the vacuum all bodies should fall in the same way.

Still though he hadn’t figured out why things fall directly below them and not half a mile away when they free fall. He kept on going with his inclined planes experiments: he saw that if a ball was allowed to roll down one plane and then back up another, the ball would reach the same height that it originally started from, and that was independent of the slope of the second plane (in a more gentle slope plane the ball would travel more distance and finally reach the same height).

This is explained today as the conservation of energy, but Galileo saw something else in it, delivering a unique piece of physical ingenuity: he claimed that if the second plane was completely horizontal, the ball would never be able of reaching its original height, and therefore it would keep moving eternally on that plane. Thus, he concluded, a body moving in horizontal motion would keep moving at the same speed forever! This idea became later on the first of Newton’s laws, the law of inertia.

Now it is evident … that the equable motion on this plane would be perpetual if the plane were of infinite extent.

Now the explanation of the falling body paradox was apparent to him: when a body falls, the earth together with the body move horizontally, and they both must maintain their horizontal velocities; therefore the body does not slow down at all with respect to earth and falls vertically down to earth. He then applied the same type of reasoning to projectiles of cannonballs near the surface of the earth, and unleashing his mathematical skills proved that it must be a parabola. At the age of 74, 1638, he published his book Two New Sciences, where he wrote about his discoveries.

The Grandmaster Takes Over: Isaac Newton

Newton (born at 1643) told at least 4 people that he was inspired by an apple tree for his theory, although this did not happen instantly and probably not by an apple falling on his head. He was always thinking on how the bodies move (“gravity” didn’t exist as a word then). He had a great rival, Robert Hooke, with whom they disagreed very often, and a great friend, Edmond Halley (the first one to observe in detail the comet which was later named after him).

When Newton was 36, they had a great debate regarding the movement of a body heading towards the center of the earth. Hooke’s persistence drove Newton at a rage. They both accepted that there was a force that was pulling heavy objects towards the center of the earth. Hooke suggested a force that depended on the distance from the center. (Halley had actually carried a pendulum to a 2,500 feet hill and judged that it swung more slowly there, supporting this claim). They just didn’t know what the power of this force was.

They both knew at the time (from Kepler) how planets were moving, and that an inverse square law might cause a body to move on an ellipse. Hooke couldn’t work the mathematics and put this to Newton straightforward: “My supposition is that Attraction always is in duplicate proportion to the distance from the Center Reciprocal”. Hooke actually managed to formulate the problem exactly: he asked Newton to find the mathematical curve and suggest a physical reason. He asked him because he knew he could work it out. He wrote to Newton about this 2 times, but he never got an answer.

In August 1684, when Newton was 41, Halley put again a question to Newton: supposing an inverse square law of attraction toward the sun, what curve would a planet make? Newton then said an ellipse: he had calculated this a long time ago (when he was 23). He started working again on it and several months passed before providing Halley with a proof.

He had great difficulties because there was no vocabulary associated with this work. So, he invented it: he defined Quantity of matter as mass, and Quantity of motion as the product of velocity and mass. He then send Halley a 9-page treatise “On the motion of bodies in orbit”. Halley was excited and asked Newton for more pages, but Newton was not finished.

Here is how James Gleick describes Newton’s finest work:

As he wrote, computed, and wrote more, he saw the pins of a cosmic lock tumbling into place, one by one. The alchemical furnaces went cold; the theological manuscripts were shelved. A fever possessed him, like none since the plague years. He ate mainly in his room, a few bites standing up. He wrote standing at his desk. When he did venture outside, he would seem lost, walk erratically, turn and stop for no apparent reason, and disappear inside once again. Thousands of sheets of manuscripts lay all around, ink fading on parchment, the jots and scribbles of four decades, undated and disorganized. he had never written like this: with a great purpose, and meaning his words to be read.

On 1686, at the age of 44, Isaac Newton gave Halley Book I of Philosophie Naturalis Principia Mathematica.

It is now established that this force is gravity, and therefore we shall call it gravity from now on.

But why was Newton’s work most important than the work of his predecessors? First, he found the laws that explained the previous discoveries of Kepler and Galileo (the laws were actually suspected, but he was the one who proved this was the indeed case). His inverse square law explained the ellipses that the planets make as Kepler realized, and his F=ma law (where F he put the newly founded gravity) explained the motion of the objects on the earth that Galileo had discovered.

Second, he generalized his theory: he realized that the same laws that apply here on earth must also apply to the movement of the celestial bodies, and this is how he managed to explain the tides: as an earthly effect of celestial bodies. He made in that way the first great unification, the first out of many that were destined to come: laws that could explain both earthly and celestial phenomena.

Newton’s work was so powerful that wasn’t altered for more than 200 years (by Einstein); his theory was the framework that included all the efforts of Copernicus, Brahe, Kepler and Galileo. He referred to them as giants, when he very eloquently said

If I have seen further, it is by standing on the shoulders of giants.

A Radical Change: Albert Einstein

Albert Einstein was 21 years old at the turn of the 20th century, 1900. By then Newton’s theory of motion gravity had dominated physics, providing the tools to explain the everyday phenomena in our world and explaining any almost physical problem imaginable. However, there were tiny discrepancies that did not fit in the theory. This was a typical case were in order to explain these small discrepancies a major breakthrough is required; a huge jump in our understanding of the world.

In terms of experiments, the astronomers had found that the orbit of Mercury around the sun is not a fixed ellipse; it moved slightly as the years passed. In Newton’s theory, this result was not predicted since all the ellipses should be fixed. It was then thought that because Mercury is so close to the huge mass of Sun, it might be affected in a gravitationally different way. Einstein managed at 1916 to find a satisfying theory that would explain this fact, but in order to get a glimpse of it we should go a few years back and look at his theory of special relativity first.

In 1905, at the age of 26, Einstein managed to extend Newton’s theory for the motion of bodies at high speeds; by assuming that the speed of light is constant no matter how one is traveling (at a constant speed) he derived an extension to Newton’s equations which holds true for every speed, not only for low ones.

Newton was still correct in that limiting case. These results however were valid only if the objects are moving at constant velocity; a replacement of Newton’s send law which describes what happens when an object is accelerated was yet to be found. Einstein was the first person responsible of extending his theory of zero acceleration to a theory of non-zero acceleration.

It took him about 10 more years to come up with an answer. During this period he realized several things: first he assumed that a person cannot distinguish whether he is in a gravitational field or under constant acceleration, i.e. these two things are equivalent. This assumption provided a link between gravity and mass, or gravity and energy. He concluded then that mass and energy must affect the gravitational field.

Secondly, he stated that the gravitational field is not actually a force as Newton has described, but instead a curvature in space. To put it in simple words, the bodies are affected by gravity not because of a force directly exerted on them but because space is curved and therefore they have to follow space’s grid. The presence of mass or energy does not affect the bodies directly; it affects the space first, and then the bodies move in this curved space. Earth always moves in a straight line (not in the Euclidian sense though). The presence of sun curves space, and therefore curves this straight line and forces earth to appear to be moving in an ellipse.

It is very difficult for an average person to realize what this really means, but I have a favorite analogy:imagine being in a sea (or pool) using your finger to create ripples on the water. The presence of your finger in the water creates these waves and alters the geometry of water, it’s not flat anymore. If you look through the rippled water you’ll see the bottom distorted.

Well, the same things happens to 3D space if you try to move your finger like that on the air! Your finger has mass and it actually can create ripples in space… The only reason you cannot see them as in the water is that the mass of your finger is so small that the ripples it creates are of the tiniest magnitude; there are so small that do not affect anything considerably.

However it has been found to be true when bigger and more massive objects such as neutron stars or black holes are carefully studied. If we were able to stick a black hole into our fingertip, then by moving the finger in thin air we would indeed see these ripples. Just the sole idea that this is already happening even at a microscopic level is at least fascinating.

This idea, that space can be bended by mass, has breathtaking ramifications. Since mass curves space and then the objects just move on this space, there is no reason why for example light couldn’t follow space’s curvature.

Indeed, one of Einstein’s first ideas was that light should be able to bend too, when massive objects exist close to it. In 1913 (before fully completing his theory) he made some interesting calculations: considering the Sun as a massive object, would the light from a star behind the sun that passes close to him be bended by its gravity?

By making some calculations, he figured that the angle which the light ray from such a star is bended is indeed measurable. He quickly send a letter to Hale at the Mt. Wilson observatory in Los Angeles and asked how could they measure this deflection. If his theory was true, then the star should appear to be in a different position that it was expected according to the astronomical catalogues.

Hale responded that since no stars are visible during daylight, the only way they could measure it was during a solar eclipse; at that time the sun’s light is blocked, and the stars in the sky are easily detected then. However the amount of bending that Einstein predicted was not correct: it was off by a factor of 2 (his theory was yet incomplete at the time).

The next scheduled solar eclipse was to take place in Russia, in 1915. Some German scientists were intrigued by Einstein’s idea, and went in Russia that summer to measure this (erroneous) deflection. However while they were in Russia World War I broke out; the German scientists were captured by the Russians and Einstein’s incorrect theory was never disproved.

(Can you imagine what would have happened if they indeed measured the wrong bending? Einstein would have proven to be wrong, and since he was not so famous by then he might never had a second chance to prove himself. Life plays interesting games, huh?).

When the war ended 2 experimental teams leaded by Sir Arthur Eddington set off to measure the light deflection at solar eclipse, at 1919. By that time Einstein had figured out the correct equations of his general relativity theory, and found the exact amount of bending. At the crucial day of the solar eclipse the two teams collected the data and then compared them to Einstein’s theoretical predictions: the matching was superb for the accuracy they had at the time.

The next day Einstein’s face was in newspapers all over the world: by predicting light bending was by itself incredible, and the confirmation made him quickly the most famous scientist ever, even to the large masses of people. Two years later we won the Nobel prize in physics (not for his work in relativity, ironically).

Towards the Future: Quantum Gravity

Einstein’s theory of gravity has never been disproved until now (2004). Soon after it’s completion, the theory of quantum mechanics was developed, a description of the world in very small scales. However general relativity seems to be incompatible with quantum mechanics and breaks down (theoretically).

In most of the cases gravity is so weak that in so small scales it is ignored. However in the interior of a black hole, the huge amount of mass is not negligible. Also, this is the case at the early stages of the universe: ultra-condensed matter, lots of mass suppressed into quantum distances. At these cases a quantum treatment of gravity will be needed, although there is no way right now to test how exactly general relativity must be modified.

The other 3 forces of nature have been modified and work well under the quantum regime, and only gravity escapes for the moment. The complete theory of gravity will include eventually somehow the quantum principles; this theory of quantum gravity is the greatest challenge of our understanding of the world today.

I wonder what the giants would think about that…